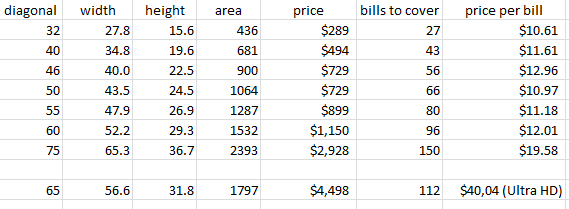Browsing the selection of huge televisions for sale at Best Buy the other day, I considered the price of the televisions compared to their screen area. The large televisions were not only more expensive, but the prices seemed to rise exponentially as the size increased. 

I had a thought that I might be able to construct a graph using ten and twenty-dollar bills to illustrate the different values for large and small screens. Before doing any actual calculations, I had a graph like this in my mind:

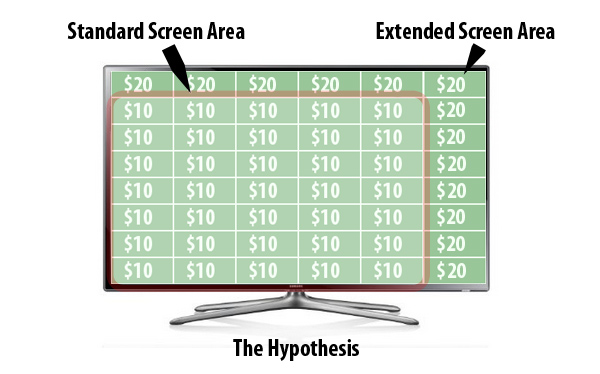
In the image above, the smaller televisions have low-priced screens when examined on a per-inch basis. The larger screens added size at a quickly-escalating cost. I thought it might even be at twice the price of smaller televisions, illustrated with a row and column of $20 bills. If you want extra space, it comes at a premium cost.

But I didn't actually know if this was accurate so I priced some televisions. The prices were all over the place, so I settled on examining the Samsung televisions on Amazon.  Here's what I found:

For a 55" tv, you use 0.489 x 0.872 x 55 x 55 and get 1289in², or 9 square feet.

Now, upon calculating the area of these two televisions, I knew my initial predictions were going to be way off. A 55" television is exponentially more expensive than a 32" tv because it is exponentially larger! 9 square feet! That's amazing, and these aren't even the largest tvs around.

Why do we use the diagonal to measure these tvs? Marketing sure didn't come up with this metric. It sounds like you aren't getting much for an additional $600, but you are getting a lot!

Hey! Dad! Over a thousand square inches! That's a marketing metric baby!

So, back to the actual dollar bill graphic. All american currency measures 6.14" wide x 2.6" tall. This equates to a very handy sixteen square inches. So, for a 32" tv, at 436 square inches, you would need 27¼ bills to cover that screen, and guess what? At $289, that's almost exactly the same value as the $10 bills laying over the area of the screen. The tv is $10 per 16 square inches, just like a $10 bill.

For the 55" tv, the increased size isn't modest. It is huge. Twenty-three more inches of diagonal translates to 857 more square inches of area.

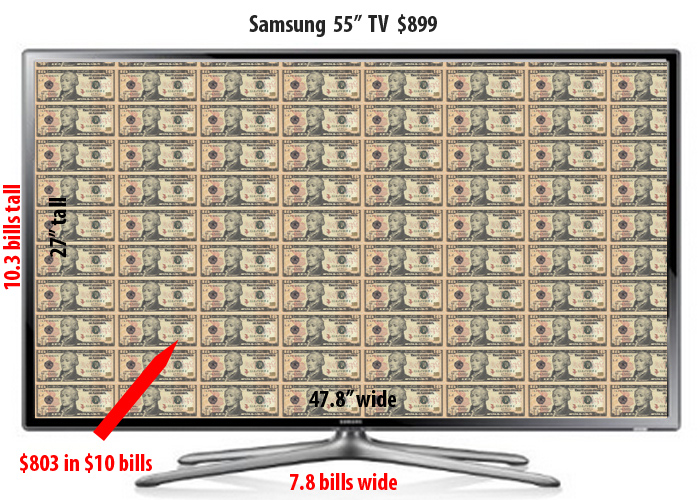
But the television is $899, $610 more than the small tv. You can't cover this with $10 bills, but you don't need $20s. If you cover the tv with $10s, it takes $803 in bills. That's $96 short.

So, what we really need here are $11.2 dollar bills. The whole tv screen is worth a cover of $11.2 bills, or we can put 27 $10s over the part of the screen from a 32" tv and cover the rest of the real estate with $11.5 bills.

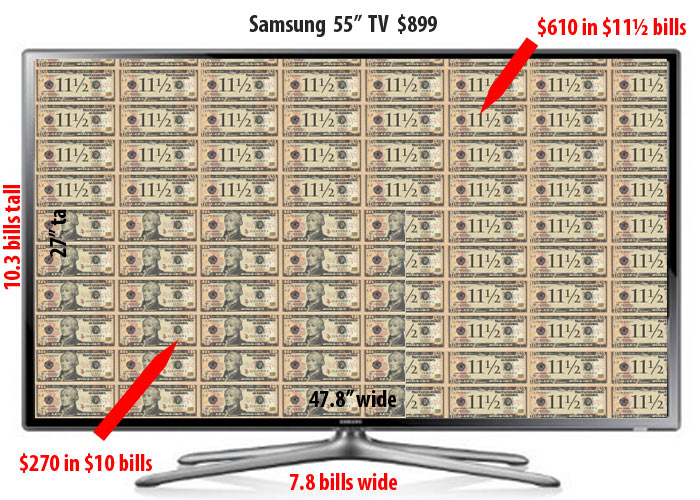
The extra screen is a little more expensive, but not that much!

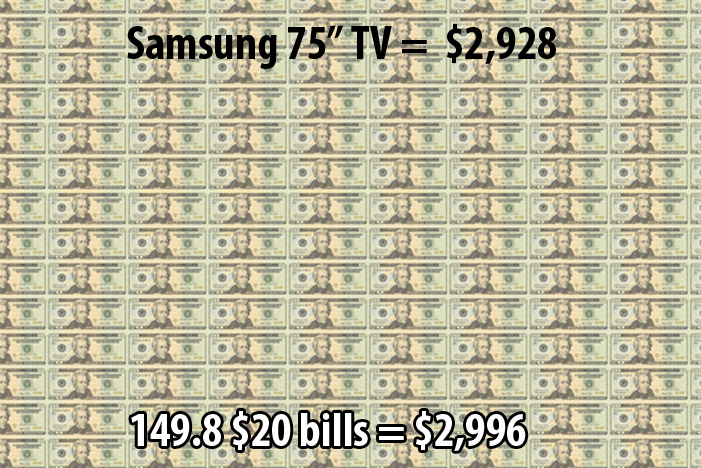
Next I calculated the size of a 75" television. It was 2,398 square inches. That's too large to show on this website without changing the scale.

If you click on the image above, the full sized television will load in a seperate page.

The 75" Samsung breaks out of the price-per-inch range of the other tvs. This screen is $2,928. That's almost exactly the same value as the $20 bills laying over the area of the screen. The tv is $20 per 16 square inches, just like the $20 bills!

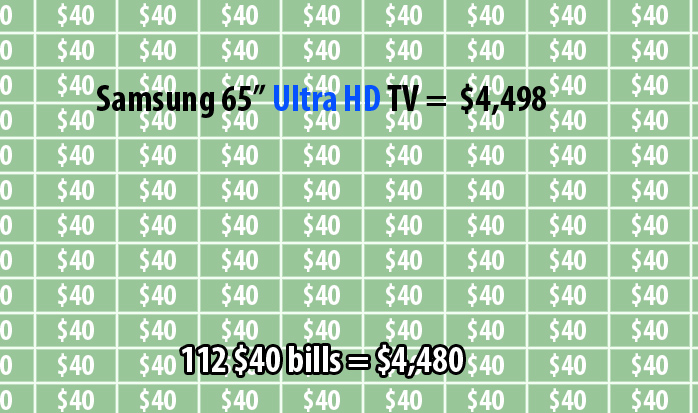
Maybe you can guess what is next, a 65" Ultra HD television! There aren't a lot of options for UHDTVs. Here's a 65" one. It is 1801 square inches in area.

If you click on the image above, the full sized television image will load in a seperate page.

Lo and behold! The Ultra HD television is almost exactly the price as a blanket of $40 bills which cover its screen exactly. Another option is to cover the screen with two layers of $20 bills.

Summary
My hypothesis was not correct. Most tv screens stay pretty much the same price per square inch, until you get to the very largest screens available. At that point, when you get to the 75" televisions, the formula I predicted is true.

To make the jump from 55" to 75", you've got to pay $30 for each extra bill-sized chunk of screen.

It's expensive, but if you've got that many $30 bills hanging around, I recommend that you buy it.

Here's the breakdown for other screen dimensions, including area, price, and the number of bills required to cover the screen.